“I remember spreading Nutella on a slice of bread and suddenly saying, ‘Jeez! That’s it!’”.
That’s how Mexican Rafael González describes the precise moment he discovered the solution to an optical physics problem that hadn’t been solved for centuries.
Isaac Newton himself couldn’t solve it in his day. Although there had been partial solutions, no-one had found the complete answer.
It was the solution to spherical aberration in optical lenses, which could now help many industries make big savings in producing items such as telescopes and cameras.
That morning, after months and months of attempting to solve the equation describing the problem, Rafael knew he’d finally got it.
“I went up to my room, began programming, saw the results and started jumping for joy”, he told CONECTA.
Rafael is currently taking a PhD in nanotechnology at Tec de Monterrey, having also graduated from its Industrial Physics Engineering program. It was his friend Alejandro’s idea for them to team up to unravel the puzzle.

Alejandro Chaparro, a UNAM graduate, had invited Rafael to solve the problem after he’d been trying to solve it himself for three years.
The pair met while studying for their Master’s Degrees at the Optical Research Center.
“I knew it was a legendary problem. That’s where I met Alejandro; he was insistent and kept asking me to help solve the problem. I told him it was a tough nut to crack and I wouldn’t be able to do it”, said Rafael.
AN ANCIENT PROBLEM
The Greek mathematician Diocles was the first person to pose the problem more than two thousand years ago.
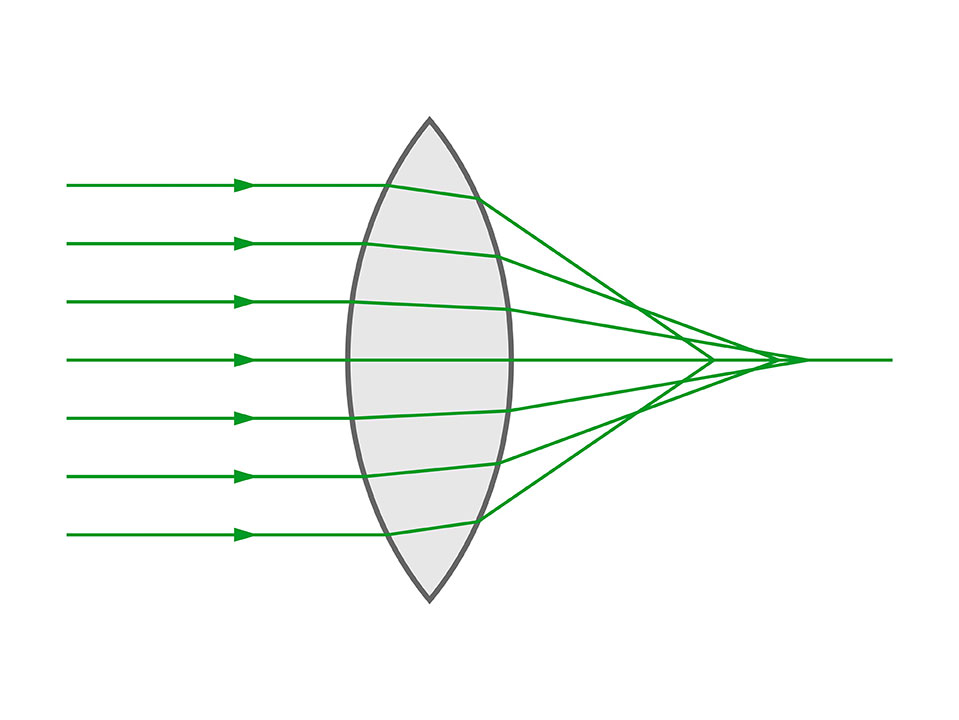
Over the centuries since, scientists such as Newton or Leibniz had taken a crack at the challenge, which is a loss of definition when viewing objects through spherical lenses.
Newton invented a telescope that solved the problem of chromatic aberration (which prevents colors from being focused on the same point), but it didn’t solve spherical aberration.
Two twentieth-century scientists formally expressed the problem in an article in 1949. From then on, the problem would be known as the Wasserman-Wolf problem.
No-one had been able to solve it completely.
Newton or Leibniz had taken a crack at the challenge, which is a loss of definition when viewing objects through spherical lenses.
THE SOLUTION AND GLOBAL RECOGNITION
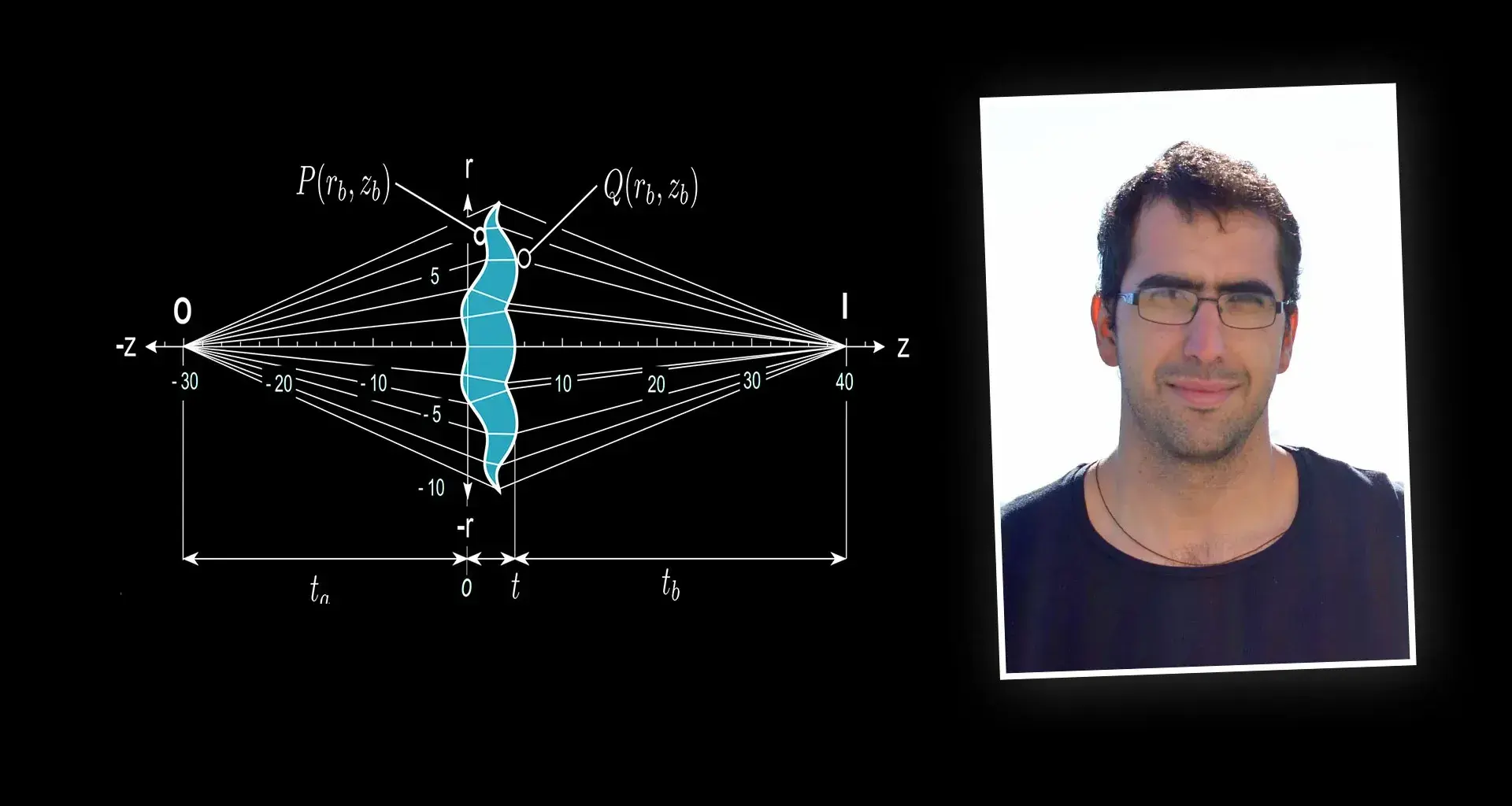
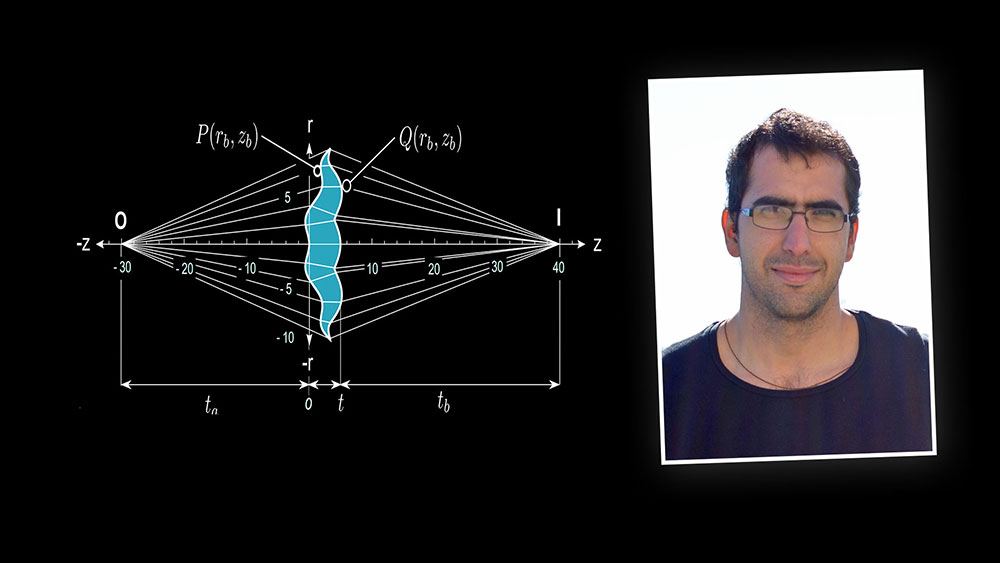
One solution to the problem was the combination of two lenses that were not spherical but aspherical (only spherical on part of their surface).
However, until now, calibrating these lenses depended on a calculation that wasn’t completely accurate.
“(By contrast) the analytical solution (discovered by them) is exact; by using the equation, you’ll get the precise result, regardless of a change in the variables”, he explained.

“We calculated the efficiency of 500 rays, and the satisfaction average was 99.9999999999%.”.
Rafael and Alejandro published the solution in the article General formula for bi-aspheric singlet lends design free of spherical aberration, in the journal Applied Optics.
“We were very fortunate, because we earned the Editor’s Pick, which is very rare: fewer than one percent of the 35,000 articles published in that journal have that distinction”, he said.
“In our study, we calculated the efficiency of 500 rays, and the satisfaction average for all examples was 99.9999999999%.”.
Equation for solving the problem of spherical aberration in lenses.
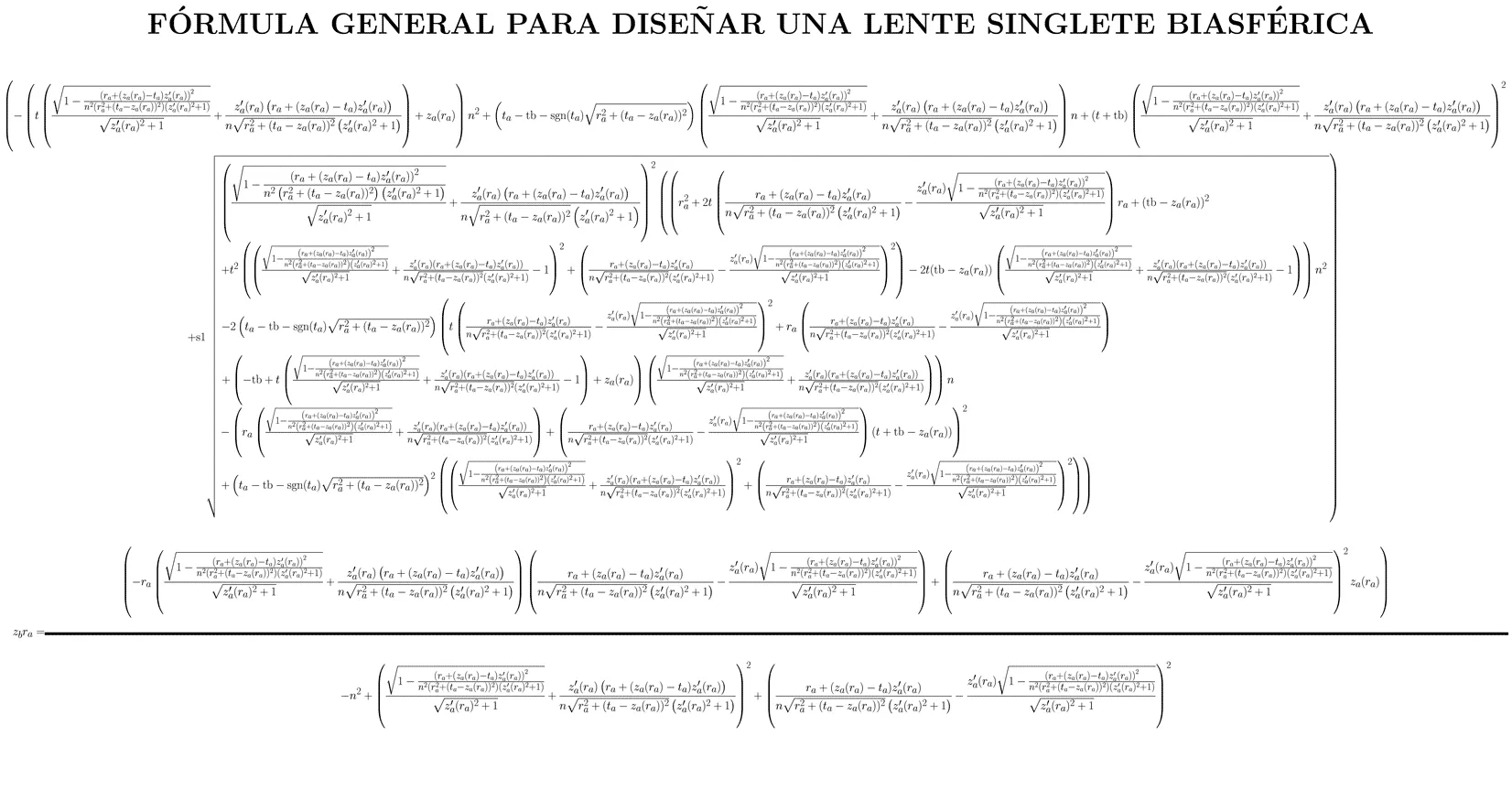
THE FORMULA’S IMPACT
Julio César Gutiérrez, the Tec professor who is currently advising Rafael for his PhD, considered that having solved the problem could imply improvements in lens development.
“The optical design has technological applications involving optical systems. So the results are not merely relevant in theory, but in other applications.
“Rafael is a very good student. He’s enthusiastic and independent. He has a lot of initiative for attempting to solve challenging problems”.
SUPPORT FROM THE TEC
At his 28 years of age, with 6 articles published in scientific journals -4 of which are on this subject- and 3 more in review, Rafael highlighted the support he had received from the Tec.
He celebrated the institution’s resources, such as support with a Mathematica software license, which he used to prepare the equations and simulations for the problem.
“However, the biggest support I had from the Tec was undoubtedly my advisor’s confidence, which pushes you to propose something and work your socks off, even though you get stuck”, he said.
Rafael is proof of that, now that he can rest after having solved this ancient problem.
“I’d been obsessed for many months”, says Rafael with a smile on his face. But now he can say: problem solved.
YOU MIGHT ALSO BE INTERESTED IN: